Case Study: The Coke Supply Chain Problem

Submitted: 20 Feb 2008

Operations Research Topics: LinearProgramming, IntegerProgramming, MasterSlaveConstraints, FacilityLocationProblem

Application Areas: Logistics, Industrial Planning, Fuel Manufacturing

Contents

• Problem Description
• Extra for Experts
• StudentTasks

Problem Description

Adapted from a real-world problem

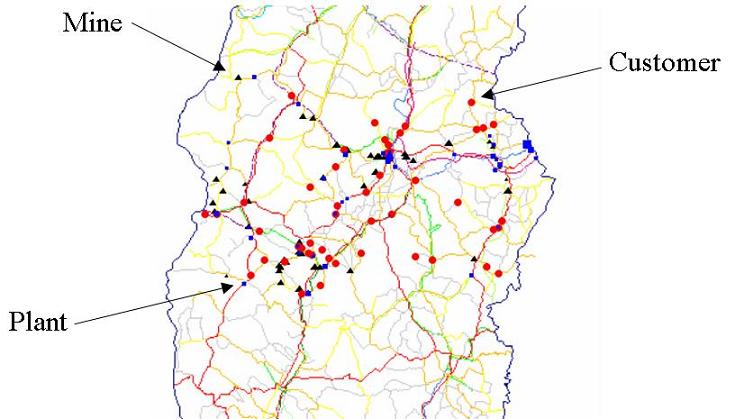
Over 29% of world's coke is made in China. Recently market liberalization has lead to town and village enterprises, with uncertainty in future markets resulting in short-sighted resource use. There are currently many small cokemaking plants with relatively primitive technology. This has resulted in an industry with low transportation costs (as coke is supplied by many small local producers) but high pollution and energy use.

Figure 1 Coke from en.wikipedia.org/wiki/Image:Koks_Brennstoff.jpg

The government wants to move cokemaking to Shanxi, where they can establish bigger facilities. This plan will be more efficient, with less pollution, but will also result in higher transport costs (calculated from a GIS database). The plants in Shanxi will be built with one or more oven batteries, each making 75,000 tonnes of coke a year. The process these plants will use to convert coal to coke is "thermal decomposition". This process results in 1 tonne of coke produced for every 1.3 tonnes of coal processed.

The (simplified) problem we will model has the following details:

1. There are six coal mines, each able to supply up to a certain amount of coal each year to the plants for processing
   
2. There are six customers, each with a certain demand for coke each year
   
3. There are six possible plant locations, each able to process a certain amount of coke each year

Each plant has 6 possible sizes, with coke processing levels and associated construction costs shown below:

Size	Cost
(kT/yr)	(MRMB)
75	4.4
150	7.4
225	10.5
300	13.5
375	16.5
450	19.6

Each Mine has the following coal supply per year:

Mine	1	2	3	4	5	6
Coal Supply (kT/yr)	25.8	728	1456	40	36.9	1100

Each Customer has the following coke demand per year:

Customer	1	2	3	4	5	6
Coke Demand (kT/yr)	83	5.5	6.975	5.5	720.75	5.5

The transportation costs (RMB/kT) between the mines and the plants are as follows:

RMB/kT	Plant 1	Plant 2	Plant 3	Plant 4	Plant 5	Plant 6
Mine 1	231737	46813	79337	195845	103445	45186
Mine 2	179622	267996	117602	200298	128184	49046
Mine 3	45170	93159	156241	218655	103802	119616
Mine 4	149925	254305	76423	123534	151784	104081
Mine 5	152301	205126	24321	66187	195559	88979
Mine 6	223934	132391	51004	122329	222927	54357

The transportation costs (RMB/kT) between the plants and the customers are as follows:

RMB/kT	Plant 1	Plant 2	Plant 3	Plant 4	Plant 5	Plant 6
Customer 1	6736	42658	70414	45170	184679	111569
Customer 2	217266	227190	249640	203029	153531	117487
Customer 3	35936	28768	126316	2498	130317	74034
Customer 4	73446	52077	108368	75011	49827	62850
Customer 5	174664	177461	151589	153300	59916	135162
Customer 6	186302	189099	147026	164938	149836	286307

Return to top

Extra for Experts

This problem is similar to the Cosmic Computers Problem, but the facility location is a little different. Rather than face a decision about whether to build a plant or not, we need to determine what type of plant to build. This gives rise to the following variable:

While solving the Coke Supply Chain Problem, the expression

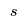
will often be fractional. This means that the capacity of the plant where is the capacity of a plant of size will be between the capacities of two different size plants, e.g., 280 kT/yr is between 225 kT/yr plant and a 300 kT/yr plant. We could use constraints to remove the fractionality, e.g.,

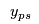
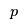
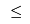
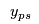
These branches will ensure plants have the right capacities, but will still not resolve fractionalities in the . However, if we want the capacity of plant to be 225, then we can remove all the that allow for the capacity to be larger than 225:

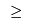
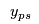
Conversely, if we want to branch up, i.e., the capacity is 300, then we can remove all the that allow the capacity to be smaller than 300:

Note For these branches to work properly, the possibility of building no plant must be modelled as building a plant with no capacity (i.e., = 0) for no cost.

Return to top

Student Tasks

1. Write AMPL model, data and script files (coke.mod, coke.dat and coke.run respectively) to solve the Coke Production Problem. Write a management summary for your solution.

   What to hand in Your 3 AMPL files. Your management summary.

2. Experts Only Solve the LP relaxation of the Coke Supply Chain Problem integer programme. Using the branches described in Extra for Experts, use AMPL to perform branching on at least 4 nodes and draw the resulting branch-and-bound tree.

   Hint See Extra for Experts in the Cosmic ComputersProblem for an example of constraint branching in AMPL.

   What to hand in Your AMPL code for implementing the branches. Your drawing of your branch-and-bound tree.

Return to top